The Fine-Tuned Universe -- the insignificance of very small
numbers
By Mark Frank
Posted December 21, 2008
Many people, especially those from the
Intelligent Design community, argue that the universe has been so finely tuned
for life that it must have been designed. This article by Rich Deem: Evidence for the
Fine Tuning of the Universe (Deem, undated) is one of thousands of similar
articles that can be easily found on the internet.
The argument is straightforward. There are
many aspects of the universe, including some of the fundamental constants of
physics, which if they had been even slightly different then life would not
have been possible. An extreme example is something called the space phase
constant of the Big Bang which according to Roger Penrose (Penrose, 2001) has
to be accurate to 1 in 101230 or the second law of thermodynamics
would not be true and life would not be possible. This is easily reinterpreted as
"the odds of this constant happening to take a value which would support life
are 1 in 101230 ". This is so enormously improbable that the
constant must have been set to this value intentionally. Hence there must be a
designer.
There have been many types of response to
this kind of argument. Some look for alternative explanations which reduce the
apparently prodigious improbability of life. They argue that the physical
constants have more leeway than supposed, or that there is an underlying
physical explanation for such constants having those precise values, or that
there are an infinite number of universes each with different values for the
physical constants and of course we are in the one that supports life.
Others treat supernatural and natural
explanations of the universe as hypotheses to be assessed using standard
techniques of statistics. They then use Bayesian logic to challenge the
conclusion that design is made more likely because the universe has some
surprising features, for example (Perakh, 2001).
These are all powerful arguments. The point
of this paper is much more limited. I want to examine if this proposition:
(A) Constant K has to be accurate to 1
in 10a very large number to support life
entails
(B) Without design, the probability of
constant K having a value that supports life is 1 in 10a very large number
Or to offer a bit more leeway, does (A)
entail
(C) Without design, the probability of
constant K having a value that supports life is very small
To keep the number of zeros down to a manageable
size I will use a different example of a fundamental constant - the ratio of
the mass of a proton to the mass of an electron -- which is just under 2000:1. This
is one of the constants that Deem identifies and he writes that life as we know
it would be impossible if that ratio differed by 1 in 1037.
The design proponent must have a picture
analogous to the following. Imagine a creator of universes with a set of dials
-- one of which is marked proton to electron mass ratio.
The creator could set the dial to a vast range of values, but with great care
tunes it to a value which sustains life. I will call the range on the dial
which includes all the values which could sustain life the life supporting range.
In the design proponent's mind the life supporting range is a tiny portion of
the dial that corresponds roughly speaking to 2000 ± 2000 * 10-37. The
alternative is that dial was twirled by some natural process and just happened
to fall in the tiny life supporting range. Hence proposition B.
Given this picture, some basic questions
arise. One is: "in the absence of design, is the dial equally likely to fall at
any point in its range or is it more likely to land in the life supporting range".
I am going to duck this one. It turns round the concept of a uniform
probability distribution and there is already a lengthy on-going dispute about this.
For the purposes of the rest of the essay I will concede that the dial is equally
likely to fall anywhere in its range.
A second question is: "what is the total range
of values on the dial?". If the life supporting range occupies 90% of the dial's
total range then the argument clearly does not hold. But how big is the non-life
supporting part of the dial's range? What are the upper and lower limits? We
have no other universes to compare and as far as I know there is no theory to
fall back on. Here are some possible answers:
- The dial's range includes every value from zero
to infinity (Maybe even from minus infinity to plus infinity. A negative ratio
is unimaginable, but in cosmology it is pretty common to talk about the unimaginable).
After all if the designer is indeed God then he is omnipotent and should be
able to set it whatever he wants. In this case it is not possible to give any
meaning to the probability of a life supporting value. You can't have a uniform
probability distribution over an infinite range. It makes no difference to the
probability whether the life-supporting range is ± 10 -37 or ± 10 +37
. We might as well forget about all the figures.
- We don't know the range of the dial. In that
case we don't know if a life supporting value is improbable or not. End of
story.
- The range of the dial is the same as the value
of physical constant in our universe.
I believe this last answer is the one that
is being implicitly assumed. It would support both propositions (B) and (C)
above. But it is an assumption which has no justification. Why should the range
of possible values have any relationship to the particular value that we
observe in our universe? It is to confuse the size of a range (a dispersion) with
a specific value within that range (a location). To give a mundane example: a
live human body is typically at approximately 310 degrees Kelvin plus or minus
one degree. This could be presented as a precision of about 0.3 per cent i.e.
less than 1 in 102. Should we then conclude that the probability of
someone having a normal temperature is less then 1 in 100? Of course not -- the
310 is irrelevant.
To say the same thing a bit more mathematically:
suppose there is random variable which is the ratio of the mass of a proton to
the mass of electron (a strange idea in itself) -- call it Μ. Its observed
value in our universe is μ. Let the range of values which would support life be
from μ1 to μ2 (of course, μ is
somewhere in this range).
So 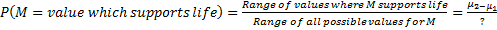
The numerator is the difference between the
largest value which will support life and the smallest. But what is the
denominator? It could the same size as μ -- which would give us a probability
of 10 -37. It could be exactly the same as the numerator which would
give us a probability of 1. It is a complete unknown. The fact that the
numerator is small compared to μ has no significance.
I appreciate that this may be counterintuitive.
The idea that a precision of 1 in 101023 has no significance feels
wrong. Surely such a mind blowingly small number must have implications. But if
there is one thing we should have learned about modern physics it is that
intuition gets us nowhere. It is world where nothing but the mathematics can be
relied upon.
Bibliography
Deem, R. (undated). Evidence for the
Fine Tuning of the Universe. Retrieved December 14, 2008, from Evidence for
God.
Penrose, R. (2001, December 30).
Retrieved December 14, 2008, from The Physics of the Small and Large. What is
the bridge between them?
Perakh, M. (2001, July 30). The
Anthropic Principles -- Reasonable and Unreasonable. Retrieved December 18,
2008, from Talk Reason.
Discussion

|


